Partial differential equations for scientists and engineers – Partial differential equations (PDEs) stand as a cornerstone for scientific and engineering disciplines, providing a powerful tool to model and solve complex phenomena. From the intricate dynamics of fluid flow to the intricate behavior of heat transfer, PDEs play a pivotal role in unraveling the mysteries of the natural world and shaping the technological advancements that define our modern era.
This comprehensive guide delves into the fascinating world of PDEs, exploring their mathematical foundations, diverse applications, and the cutting-edge computational techniques employed to solve them. Prepare to embark on an enlightening journey that will equip you with a profound understanding of these equations and their indispensable role in shaping our scientific and technological landscape.
1. Definition and Overview: Partial Differential Equations For Scientists And Engineers
Partial differential equations (PDEs) are mathematical equations that involve unknown functions of multiple independent variables and their partial derivatives. They play a crucial role in scientific and engineering fields, providing a framework to model and analyze complex physical phenomena.
PDEs arise in various disciplines, including fluid dynamics, heat transfer, wave propagation, electromagnetism, and quantum mechanics. They allow scientists and engineers to describe and predict the behavior of systems by relating the changes in a dependent variable to changes in multiple independent variables.
2. Mathematical Concepts
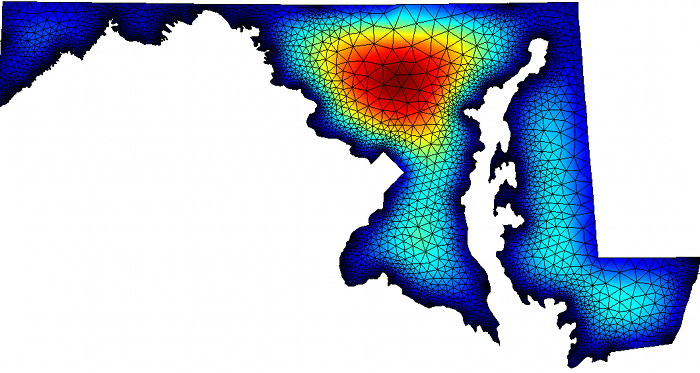
PDEs are classified based on their order (highest derivative order) and linearity (whether the unknown function appears linearly). Common types include first-order PDEs, second-order PDEs, and nonlinear PDEs.
Solving PDEs involves applying mathematical techniques such as separation of variables, method of characteristics, and Fourier analysis. Numerical methods, such as finite difference, finite element, and spectral methods, are often employed to approximate solutions when analytical methods are not feasible.
3. Applications in Science

PDEs are widely used in physics to model fluid flow, heat transfer, and wave phenomena. In chemistry, they are employed to study reaction-diffusion processes and transport phenomena. In biology, PDEs are used to model population dynamics and disease spread.
By solving PDEs, scientists can gain insights into the behavior of complex systems and make predictions about their future states. For example, PDEs are used to predict weather patterns, simulate fluid flow in aircraft engines, and model the spread of infectious diseases.
4. Applications in Engineering
PDEs are extensively used in engineering to design and analyze structures, fluid systems, and electrical devices. In mechanical engineering, PDEs are employed to model stress and strain in materials, fluid flow in pipes, and heat transfer in engines.
In electrical engineering, PDEs are used to analyze electromagnetic fields, design antennas, and simulate the behavior of electronic circuits. By solving PDEs, engineers can optimize the performance and safety of engineered systems.
Questions and Answers
What are partial differential equations?
Partial differential equations (PDEs) are mathematical equations that involve partial derivatives of an unknown function with respect to multiple independent variables.
What are the different types of PDEs?
PDEs can be classified into various types based on their order, linearity, and the number of independent variables involved.
How are PDEs used in science and engineering?
PDEs are extensively used in science and engineering to model and analyze complex phenomena, such as fluid flow, heat transfer, and wave propagation.
What are the common numerical methods for solving PDEs?
Common numerical methods for solving PDEs include finite difference, finite element, and spectral methods.
What software tools are available for solving PDEs?
Numerous software tools are available for solving PDEs, such as MATLAB, COMSOL, and ANSYS.